DZJ-135 Technical Parameter Information
- Eccentric Moment:
- 806 NM
- Centrifugal Force:
- 0-883 KN
- Max. Line Pull:
- 420 KN
- Max. Amplitude:
- 0-13 MM
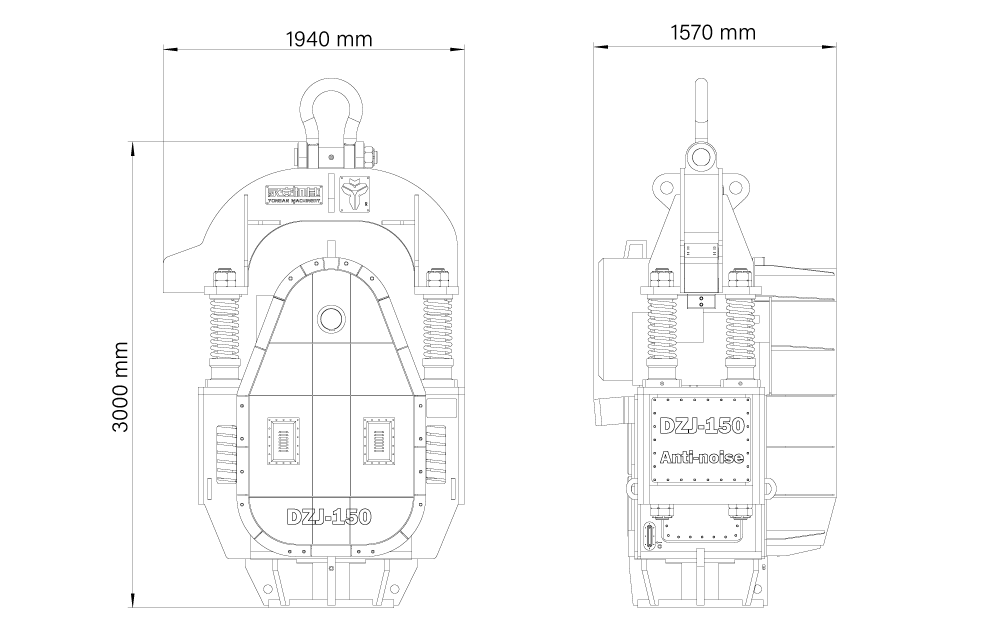
- Dimensions (w/o clamp L*W*H):
- 2100x1450x3000 mm
- Weight (w/o clamp):
- 7200 kgs
- Fitable Clamp Type:
- Single / Double Clamp
- Motor Power:
- 135 KW
- Frequency:
- 0-1000 rpm
- Electric Cable:
- 70 mm²
- Scope for double clamp:
- 600-2000 mm
- Generator Capacity:
- 300 KW
- Crane Capacity Required:
- 70 Ton

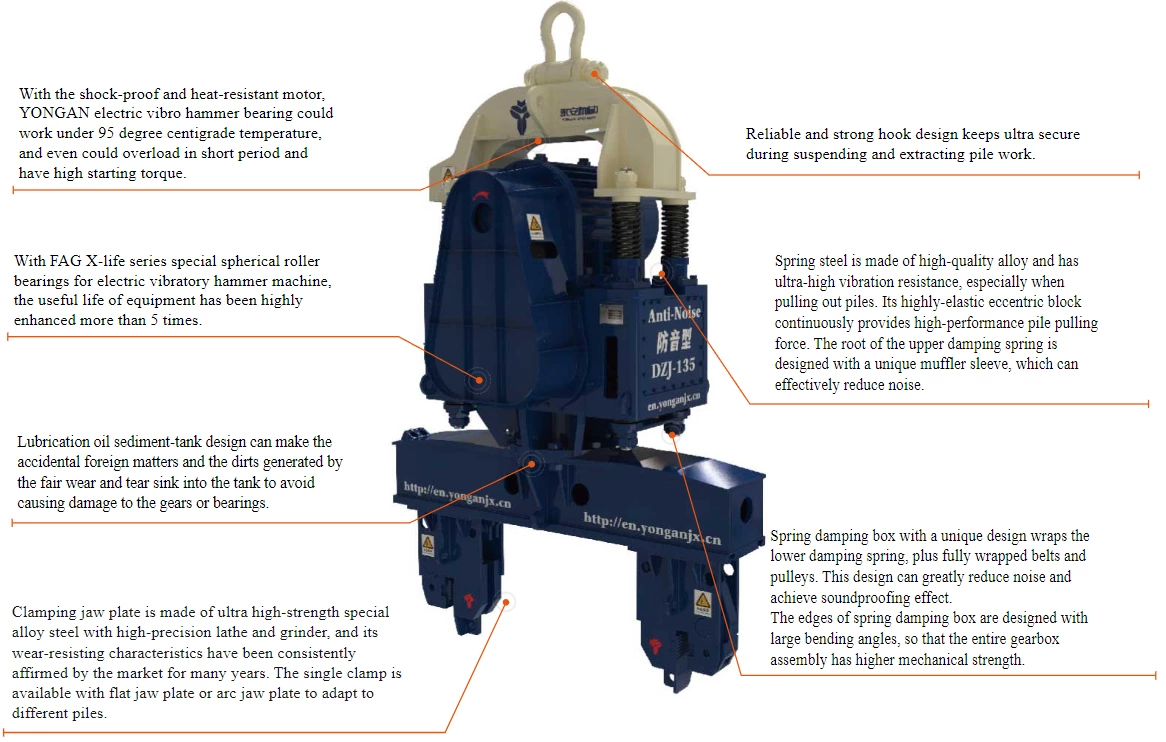
Feature1: Anti-vibration design electric motor
Yongan Vibratory hammer using the anti-vibration design electric motor, the bearing could working upto 95 degree temperature, nd could overload in short period of time and high torque, wedge shped belt is high strengt.
Feature2: FAB X-life bearing
The bearing of the vibratory hammer required receive impact load, radial accelerate, high limiting speed in a limited space, and also need to carry non-concentricify, deflection curve etc. additional oad. the traditional cylindrical roller bearing is not able to meet such requirement. In that case, we are using X-life high quality bearing from FAG. X-Life series could carry 18% more load and increase 70% of the bearing life.
Product Description
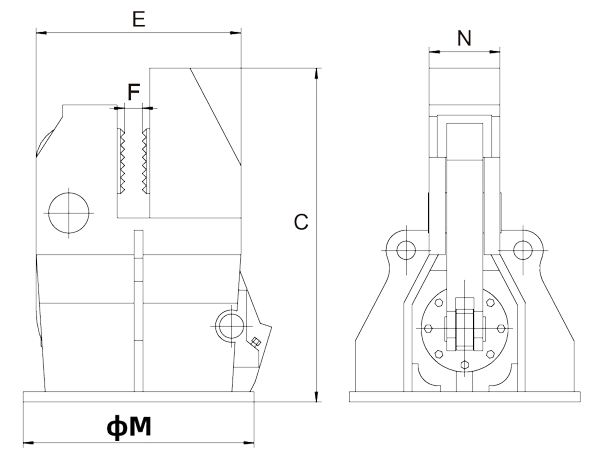
Products Size
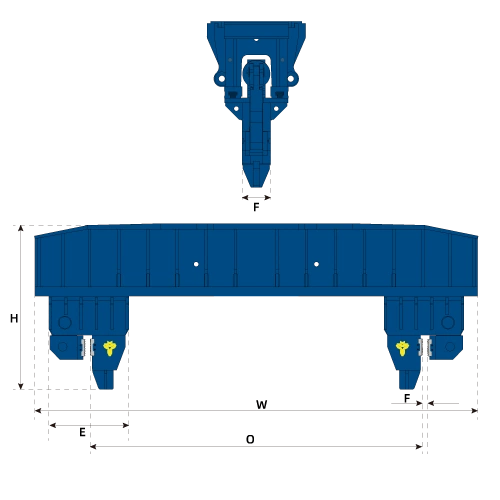
| Model No. | Clamping Force (kN) |
H (mm) |
W (mm) |
O (mm) |
N (mm) |
E (mm) |
F (mm) |
Weight (KG) |
Suit for Hammer Model |
|---|---|---|---|---|---|---|---|---|---|
| ZYJ-II480X2 | 960 | 1245 | 2500 | 600-2000 | 250 | 620 | 0-48 | 3300 | DZ-90/DZJ-90/DZJ-120 |
| ZYJ-II1050 | 1050 | 1390 | 3100 | 600-2500 | 250 | 720 | 0-50 | 3700 | DZJ-135 |
| ZYJ-II1300X2 | 2600 | 1670 | 3500 | 800-3000 | 250 | 750 | 0-55 | 3820 | DZJ-180/DZJ-200/DZJ-240 |
| Model No. | Clamping Force (kN) |
C (mm) |
N (mm) |
E (mm) |
F (mm) |
ΦM (mm) |
Weight (KG) |
Suit for Hammer Model |
|---|---|---|---|---|---|---|---|---|
| ZYJ-I75A | 750 | 1100 | 190 | 670 | 0-50 | 540 | 600 | DZ-45 |
| ZYJ-I75B | 750 | 1100 | 190 | 670 | 0-50 | 650 | 600 | DZ-60 |
| ZYJ-I85 | 850 | 1130 | 250 | 690 | 0-50 | 720 | 1100 | DZ-90/DZJ-90 |
| ZYJ-I130 | 1300 | 1375 | 250 | 790 | 0-50 | 780 | 1500 | DZJ-120/DZJ-150/ DZJ-135 |
Mounting Methods for Vibro Hammer
Crawler Crane
Crawler Rig Lead support
Rough Terrain Crane

Floating Crane
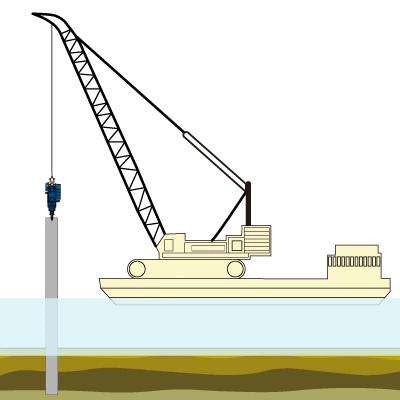
Crane Fitted Barge

Piling Barge
Electric Vibro Hammer Working Principle
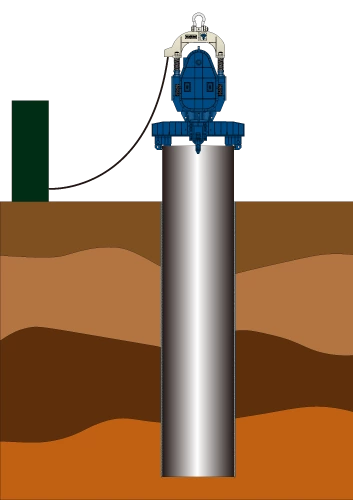
Working Principle:
The vibratory piling hammer is designed using the principle of resonance theory. When the forced vibration frequency of the pile is consistent with the vibration frequency of the soil, the soil will resonate accordingly. At this time, the soil condition and the pile sinking resistance, especially the side resistance between pile wall, will decrease rapidly, and the pile will sink under its own weight to complete the vibratory piling work.
Since the vibratory piling hammer achieves the purpose of pile sinking by reducing the friction between the pile wall and the soil, when the friction between the pile wall and the soil is reduced, a force slightly greater than that of the pile and the pile body can be used to reduce the friction between the pile wall and the soil. The pile is pulled up.
Compared with the impact piling hammer, the vibratory piling hammer has the functions of piling and pulling at the same machine, so it has been more and more used in recent years.
Vibro Hammer Main Parameters Explain:
Figure out Amplitude A:
The larger the amplitude is, the faster the pile sinks. The Pile will not sink when the amplitude is very small.
The pile will only sink when the amplitude reaches certain degree.
The value at this point is called the start amplitude A0.
With the increase of amplitude, the pile sinking speed also increased accordingly, until tend to limit value of Ac electric power.
This is called amplitude ranges. So the amplitude range is A0 < A < Ac. The initial amplitude A0. So the standard value of penetration of soil N can be applied as: A0≥N/12.5-3(mm).
Figure out Centrifugal Force F:
Centrifugal force F is the most important parameter for a vibratory piling driving hammer, and it reflects the parameters of the comprehensive piling ability of the vibratory hammer.
The centrifugal force F must be greater than the static friction force f between the pile wall and the soil, and it will drop sharply under the action of the driving pile force during the pile sinking process.
When there is vibration, the friction between the pile and the soil is denoted by f'. Expressed by the formula: F≥F' =μf(kN).
M is the reduction coefficient of friction when vibration is applied, which is mainly governed by the magnitude of vibration acceleration.
Experiments show that the vibration acceleration exceeds 10 times the acceleration of gravity. The change of μ is very small, These two values μ and f'tend to be constant.
Figure out Eccentric Moment M:
The greater the eccentric moment M of a vibratory pile driving hammer, the stronger the ability of the pilling hammer to overcome the hard soil layer.
When the amplitude A and the parameter total weight Q are known (the total weight of the pile body plus the total weight of the vibrating hammer).
According to these known parameters, we can calculate the eccentric moment required for piling according to the following formula:
M=Q.A(Nm)
Figure out Frequency ω:
The vibration frequency of the vibratory pile driving hammer is closely related to the inherent frequency of the vibration system.
When the vibration frequency is close to the inherent frequency of the vibration system, the vibratory pile sinking achieves the maximum effect.
The inherent frequency of the vibration system is not only related to the parameters of the vibratory hammer, but also related to the parameters of the soil.
There are huge differences in the natural frequencies of soils in different strata.
The following table is the best frequency range of vibrating hammers in different formations obtained from years of construction experience.
Reference for Centrifugal Frequency
| Soil Layer | Best Frequency |
|---|---|
| Saturated Sand | 100-200 ω/s |
| Plastic Clay Soil and Sandy Clay Soil | 90-100 ω/s |
| Hard Clay Soil | 70-75 ω/s |
| Gravel Clay Soil | 60-70 ω/s |
| Sandy Gravel Soil | 50-60 ω/s |
Soil Penetration standard value
| Soil Type | N value |
|---|---|
| Very Loose Sandy Soil | 0-4 |
| Soft Clay Soil | 2-4 |
| Loose Sandy Soil | 4-10 |
| Medium Hardness Clay Soil | 4-8 |
| Medium-Density sandy soil | >10-30 |
| Hard Clay Soil | 8-15 |
| Strong Density Sandy Soil | 30-50 |
| Very Hard Clay Soil | 15-30 |
| Very Strong Density Sandy Soil | >50 |
| Extremely hard clay soil | >30 |
Experiments show that under certain other parameters, increasing the vibration frequency can accelerate the liquefaction of saturated sand, and the soil resistance will be reduced accordingly. Compared with increasing the Amplitude A, it can effectively increase the movement acceleration of the pile, thereby significantly improving the pile sinking efficiency, But increasing the vibration frequency too high will cause the output power to be too large, so comprehensive consideration should be given to the determination of the vibration frequency.
Figure out Participant Weight Q:
In addition to the necessary amplitude and acceleration, the vibratory hammer must also have a certain vibration weight to overcome the resistance of the pile.
The static resistance R of the pile in the soil and the penetration value of the soil layer N and the pile end tip cross-sectional area S The relationship between is: R=4N.S (kN),
therefore, when the pile is subjected to vibration and the friction is significantly reduced, the pile can be sunk to the pile end resistance with the same vibration weight, The Formular is, Q=4N. S
Figure out Vibrating Power N:
The formula as follow: N=K.M.n/9550 in formular, n refers to the vibro hammer frequency; K=1.25
Piling Design methods and dynamic bearing capacity by vibro hammer methods
֎ Designing
Based on a large number of load test results on piles, the ultimate bearing capacity (Ru) of steel pipe piles, which are driven using a vibro hammer, is calculated by a maximum of 300 kN/m2 bearing capacity of the point, according to the pile diameter to the embedment length for the same bearing layer as the impacthammers, and pile friction resistance of all types of soil condition.
֎ Dynamic bearing capacity
The formula of dynamic bearing capacity for vibro hammer methods, which is a dynamic pile driving method, has been created and adopted in many actual applications to check the dynamic bearing capacity as a way of the pile driving control.
The formula is an extremely reliable tool in reaching the bearing capacity by the pile displacement velocity, total power consumption (in kilowatts) of the vibro motor, the characteristics of the piles being used, and soil conditions of construction.
֎ Bearing Capacity Formula

֎ Dynamic Bearing Force
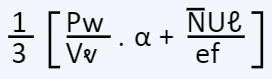
֎ Explain
Ru: Critical bearing force decide by the ground (k/N)
A: Tip of the pile blockade area (m²)
qd:The point bearing force degree
U: Surrounding length of the pile (m)
ℓi: Thickness of the layer considering the groundside friction force(m)
fi: Maxim round side friction force of the layer considering the round side friction(kN/m²)
Ra: Allowable bearing capacity
Pw: Total consumption electricity (KW) of the motor
Pw=1.3 X IA X E10-³
Ia: Electric Current(A)
E: Voltage(V)
Vⱴ: Displacement velocity (cm/sec)
N: Average N value against the surface area
ℓ: Length of the pile driven into the ground
ef: Revised coefficient
Piling Project of DZ-45 DZ-60 Electric Vibro Hammer















